How Many Useful Pickup Combinations Can You Get From 5 Coils?
© 2016 Don Baker dba android originals LC
How many possible connections, musically meaningful or not, can you get from 5 electromagnetic coil pickups? More specifically from two humbuckers, one each at the bridge and neck, with a single coil in the middle. If you are going to sell something based upon the many choices you offer, what choices in what order did you pick to offer, and what musical sense to they make? For a simple example, a clear progression from warm to bright, like a tone pot.
There’s a simple calculation for how many unique combinations you can get of N things taken M at a time, without regard for order of choice: N!/(M!*(N-M)!), where N! = N*(N-1)*…*2*1. So if you take 5 pickups 2 at a time, you get 5!/(2!*3!) = 10. Note that the 3! term cancels out of the numerator and it can be written as (5*4)/(2*1) = 10.
You can pretty much figure that any odd number of coils is going to have hum. If you restrict your choices to humbucking, only the even number of coils matter. And BTW, if you do contra-phase right, it can be just as rich and bright as any single coil at the bridge. But just for the sake of argument, let’s try to find as many musically meaningful combinations as possible, hum or not.
For 5 coils taken 1 at a time, there are only 5/1 = 5 unique combinations, all producing hum. For 5 coils taken 2 at a time, there are 10 unique combinations. Does order matter? No, but the topologies of connections do. Two pickups can only be connected together in two different topological electric circuits, serial and parallel, as shown below.
 It makes no electrical difference in the signal if pickups A and B are switched in position in the circuit. Because of the differences in magnetic polarity and phase, it does make a difference if the connections of A are reversed or B are reversed, but we’ll pass on that for now, assuming that the connections will be made in whatever way best cancels hum, whether it produces an in-phase or contra-phase signal. So now we have 5 coils taken two at a time with 2 different topologies and no order (or one order only), for 5*2*2 = 20 unique tonal combinations, all capable of humbucking if connected correctly. As noted previously, using two humbuckers and a single coil pickup reduces the number of useful humbucking pairs to 16, because the two coils in each humbucker are so close together.
It makes no electrical difference in the signal if pickups A and B are switched in position in the circuit. Because of the differences in magnetic polarity and phase, it does make a difference if the connections of A are reversed or B are reversed, but we’ll pass on that for now, assuming that the connections will be made in whatever way best cancels hum, whether it produces an in-phase or contra-phase signal. So now we have 5 coils taken two at a time with 2 different topologies and no order (or one order only), for 5*2*2 = 20 unique tonal combinations, all capable of humbucking if connected correctly. As noted previously, using two humbuckers and a single coil pickup reduces the number of useful humbucking pairs to 16, because the two coils in each humbucker are so close together.
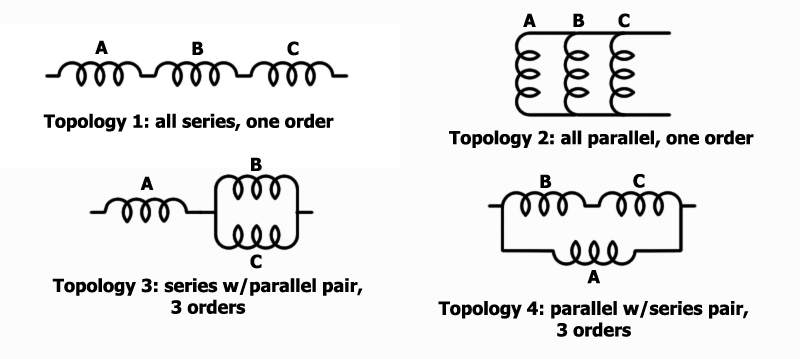
For 5 coils taken 3 at a time, or (5*4*3)/(3*2*1) = 10, I found four 3-coil topologies shown below: all serial, all parallel, one in series with a parallel pair, one in parallel with a series pair.
Order does not matter in the first two, nor in the parallel or serial pairs in the other two. For example, it does not matter electrically if you interchange B with C in the Topology 3 drawing. But it does matter if you interchange B or C with A. That gives the three orders, with A, B and C in the A-position in topology 3. The same for topology 4. This means 8 different configurations where topology and order matter. So 10*8 = 80. With hum.
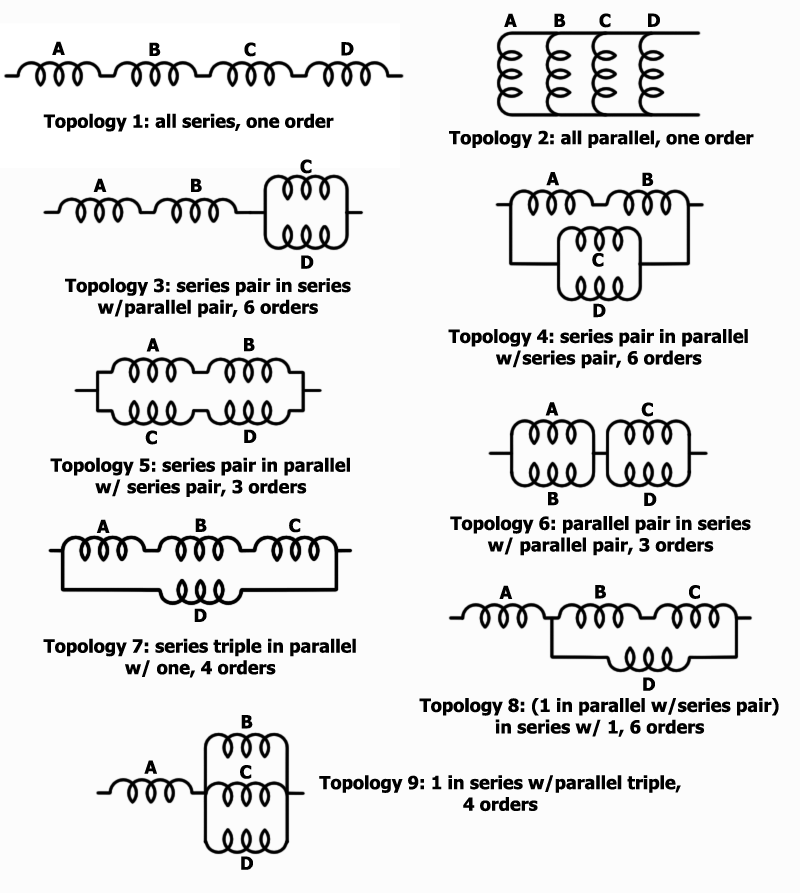
For 5 coils taken 4 at a time would have just 5 combinations, and a lot more topologies. I found 9 different electrical topologies, shown below, but may have missed something.
In topologies 1 and 2, order does not matter. In topologies 3 and 4, order does not matter within each serial pair and each parallel pair, but the combinations of AB, AC, AD, BC, BD and CD do, but only in one of the pairs, because the same combinations in the other pair are mirror images or repetitions. In other words, AB in the series pair produces the same result as CD in the parallel pair. So the combinations are 4 things taken 2 at a time, 4*3/(2*1) = 6. In topologies 5 and 6, mirroring means that the only combinations which are unique are AB, AC and AD in one of the pairs. In topology 7, the D position controls the number of combinations, leaving just 4 orders, with D, A, B and C in that position. The same thing occurs in topology 9, with 4 orders. Topology 8 is controlled by the A and D positions, 4 things taken 2 at a time, or 6 different orders.
So for 5 pickups chosen 4 at a time, there are (1+1+6+6+3+3+4+6+4) = 34 orders, making ((5*4)/(2*1))*34 = 340 unique combinations. We can safely suppose that topologies 1 to 6 are humbucking. Not so much for topologies 7 to 9. That means 10*(1+1+6+6+3+3) = 200 safe choices out of those 340. It’s not so easy to say that all those choices sound different. Not without testing and verification. The mix of in-phase and contra-phase pairs will likely make the most difference. And the switches necessary to produce these combinations probably won’t be cheap.
5 coils taken 5 at a time has just 1 unique combination, but I didn’t even try to figure out how many topological variations are possible, as I don’t have any systematic way to produce solutions. But 5 in series should be about 5x the volume of 5 in parallel, maybe. And I don’t doubt they all have some hum.
Staying with safe humbucking choices of pairs and quads, that leaves 220 choices. Using 2 standard humbuckers for the five coils cuts those down to about 16 choices of humbucking pairs with possibly unique timbres. The number of possibly unique humbucking quads of that 200 is left as an exercise for the reader. Even if all those choices have different timbres, that’s probably more choices that most guitarists would care to fool with. Unless you can create an order to the switching that makes musical sense in musical theory (which I don’t have).
In my 2014 patent, I put an extended fret scale to the sides of the movable pickups to assist any calculations of musical theory. The neck pickup sits at the 24th virtual fret, the next is 5 frets down from that, the next 7 frets down from that, and the bridge 12 frets down from that, which turns out to be almost equal spacing. If you pluck a fundamental, you might be able to say something from those spacings about the timbre of the result. But that changes in ways I don’t understand as soon as you finger another fret.
Furthermore, the way you pick the string makes a difference. I’ve watched strings vibrate in an elliptical orbit (about the relative position of the string at rest), with a rotating major axis that changes amplitude over time in relation to the minor axis. You can see it going from a flat vibration at an angle to the guitar top to a full circle and back. The results of which you can even see in the pickup signal shown on an oscilloscope. I’ve seen strings picked carefully produce and almost perfect triangle wave (well, maybe that was with a non-magnetic sensor). But the research papers I’ve seen tend to reduce string vibration to simple Fourier components, without ever touching on this behavior. I think it’s still an open question.
Even the calculations here are open to some debate, but only on the merits of the math. If you can demonstrate that I’ve missed something by the math (entirely possible), you win – three points in the competition dunking for toilet paper.