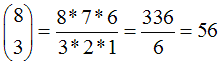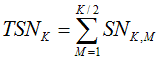© 2016 Don Baker dba android originals, TulsaSoundGuitars.com, HumbuckingPairs.com
Mathematical Definitions
First we need some definitions of mathematical terms to bring everyone onto the same page.
Factorial n: the product of all numbers from n down to 1
Math 1: n! = n*(n-1)*(n-2)* … 2*1, i.e., 4! = 4*3*2*1 = 24
Summation series: the sum of a set of expressions with the same formula, differing according to the incremental change (usually an integer change) of one or more terms. Such as, the summation of n! from 1 to 4, by increments of 1:
Product series: the product of a series of expressions, which change according an increment of one of the terms, such as the product of i as i decreases from n to 1:
Permutations: the number of unique ways to separate k things out of n things, if the order of which thing comes first matters:
For example, the number of ways can 8 Olympians be awarded gold, silver and bronze is 8!/5! = 40320/120 = 336. Note the two notations (of several) shown above for permutations. We are not really going to use permutations, but it helps to understand combinations.
Conbinations: the number of unique ways n things can be taken k at a time without regard to order:
For example, the number of ways that 8 Olympians can stand on the podium, regardless of who wins what is 336/3! = 56. Another way to think of it is to put 3 factors in the top and bottom of a fraction:
Unique combinations of KK dual-coil humbucking pickups taken MM at a time, NKK,MM
Combinations of pickups don’t tell you how to connect them together. They only tell you how many you have to work with. In this case, the formula is simple:
For example, if you have 3 humbuckers, and you want to know how many pairs of humbuckers (the equivalent of a humbucking quad of matched single-coil pickups) you can make out of them, the answer is:
The total number of all possible different unique combinations, from MM = 1 to KK is:
In other words, for 3 humbuckers, the number of single, pairs and triples (equivalent to pairs, quads, and hexes of matched single-coil pickups) is:
Table 1 shows how the number of combinations fall out for 1 to 6 dual-coil humbuckers. Note that the columns in the table are binomial coefficients, and the bottom row follows a progression, 1, 1+2, 3+4, 7+8, 15+16, 31+32. So the table could be extended indefinitely in that manner.
Although the number of PAF-sized humbuckers that can fit between the neck and bridge of a guitar is probably no more than 4, a piano would have a lot more room.
Unique combinations of K match single-coil pickups taken M pairs at a time, SNK,M
This is a bit more tricky. At the humbucking pair level, dual-coil humbuckers are already fixed. If you have humbuckers AB, CD & EF, for the inner coils A, B, C, D, E & F, coil A is always connected to coil B. But for the matched single-coil pickups, A, B, C, D, E & F, any coil can associate with any other coil.
For the coil set A & B, we have a single pair, AB, or the number of the combination of 2 things taken 2 at a time. For the coil set A, B & C, we have the pairs AB, AC & BC, or the number C(3,2) = 3. For the coil set A, B, C & D, we have the pairs, AB, AC, AD, BC, BD, and CD, or the number C(4,2) = 6. We also have the unique quad sets, (AB,CD), (AC,BD) & (AD, BC), the number of 3. This is obiously not the same as 6 pairs taken 2 at a time, C(6,2) = 15. It can’t be because if we had a combination like (AB,AC), it would not be a quad, and there is no way it could be humbucking.
So what happens if we add a 5th coil, E? First, all the combinations from the first four still exist. To those we add the combinations derived from adding E. E can combine uniquely with each of the original 4 pickups for form K-1 pairs additional pairs, for a total of 10. To form quads, the remaining 3 pickups can be taken 2 at a time to form the second pair, for a total of 3. So E adds 4*3 = 12 humbucking quads for a total of 15.
If we add a 6th coil, F, We have not only more pairs and quads, but a humbucking hex, (AB, CD, EF). If we add a 7th coil, G, we have more pairs, quads, and hexes. If we add an 8th coil, H, we have more pairs, quads, hexes, and a humbucking oct.
Without going through the entire development, the equation that results for the number, SNK,M, of unique combinations of K matched single-coil pickups organized as M humbucking pairs is:
Note that in the last form, (j-2M)! need not be calculated, but simply removed from the sequence of the product (j-1)!.
As before with dual-coil humbuckers, the pairs, quads, etc., for a given K can be added together to get the total number of humbucking combinations of matched single-coil pickups, TSNK.
Again, this does not tell us how to connect the pickups together, only how many humbucking pairs, quad, hexes, octs, etc., of electromagnetic pickups there are to work with.
Published Aug 5, 2016. Correction: Table 2 was calculated in a spreadsheet, and indicated errors in the previously posted page in HumbuckingPairs.com. Those have been corrected.
Aug 6, 2016 Note: It’s possible for this math to contain errors. Math 11 has been reworked. If you find any, please let me know, mailto: axe1 [a] TulsaSoundGuitars [d] c*m No solicitations for web site development or mobile apps, please. I do my own work.